Why should you play Tangrams with your children? They look so simple! A bunch of shapes assembled and reassembled to form newer ones! But don’t be fooled, they are not easy. Tangrams offer children an excellent opportunity to test out different geometric manipulations and become familiar with the properties of the shapes they use.

**********

This original tangram tale is framed by the loving relationship between a grandfather and granddaughter as they share the story under the shade of an old tree. Here’s a folktale with a twist: the author uses tangrams, a traditional “visual aid” employed by Chinese storytellers, to spin a tale about two shape-changing fox fairies. Seven “tans” (standard-sized pieces of a square) are arranged and rearranged to represent various characters in the story. The fox fairies vie to outdo each other–the first one becomes a rabbit, the other a dog who chases him, and so on–but when the two chase each other right into danger, they finally have to set their competition aside and pull together.
Listen to the story
**********
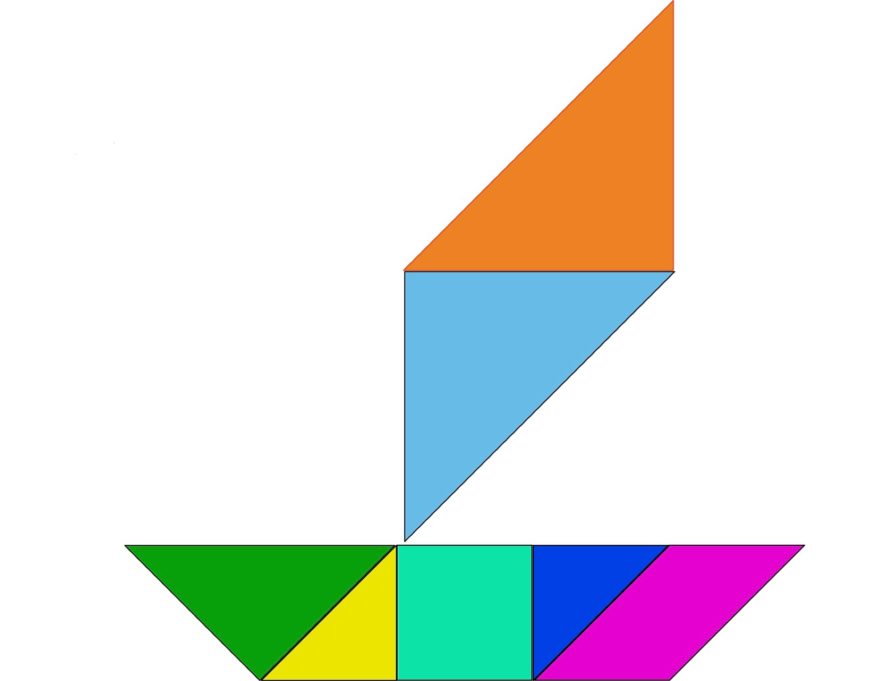
TANGRAMS

The tangram is a puzzle consisting of seven flat shapes, called tans, which are put together to form shapes. Each Tangram puzzle contains; 5 isosceles triangles of various sizes (2 large congruent triangles; 1 medium-size right triangle; 2 small congruent triangles) 1 square, 1 parallelogram.The objective of the puzzle is to form a specific shape (given only an outline or silhouette) using all seven pieces, which may not overlap. It is one of the most popular puzzles in the world. Tangrams are an excellent way to increase mental and visual skills. Using imagination, geometrical shapes, letters, numbers, figures, boats, animals and objects can bring great fun along with a challenge.
TANGRAM “HOW TO” VIDEO
**********
Activities
YOUNGER CHILDREN –Work together to create these 2 shapes


**********
OLDER CHILDREN – TANGRAM CHALLENGES
Rabbit #1
Rabbit #2

Parents: University of Cambridge article about Tangrams, “The Development of Spatial and Geometric Thinking: The Importance of instruction”. https://nrich.